The Kuta software – infinite calculus evaluating limits worksheet answers is a valuable resource for students studying calculus. This worksheet specifically focuses on the important concept of evaluating limits at infinity. Here, we will explore the benefits of using this worksheet and how it aids students in mastering this calculus concept.
Calculus can be a challenging subject, and one of its crucial concepts is evaluating limits, especially at infinity. The Kuta Software worksheet is designed to help students tackle this complex topic effectively.
Before we dive into the worksheet, let’s establish a strong foundation by revisiting the basics of evaluating limits. A limit represents the value a function approaches as it gets closer and closer to a particular point.
infinite calculus evaluating limits worksheet answers- (Paper 1)

infinite calculus evaluating limits worksheet answers- (Paper 2)
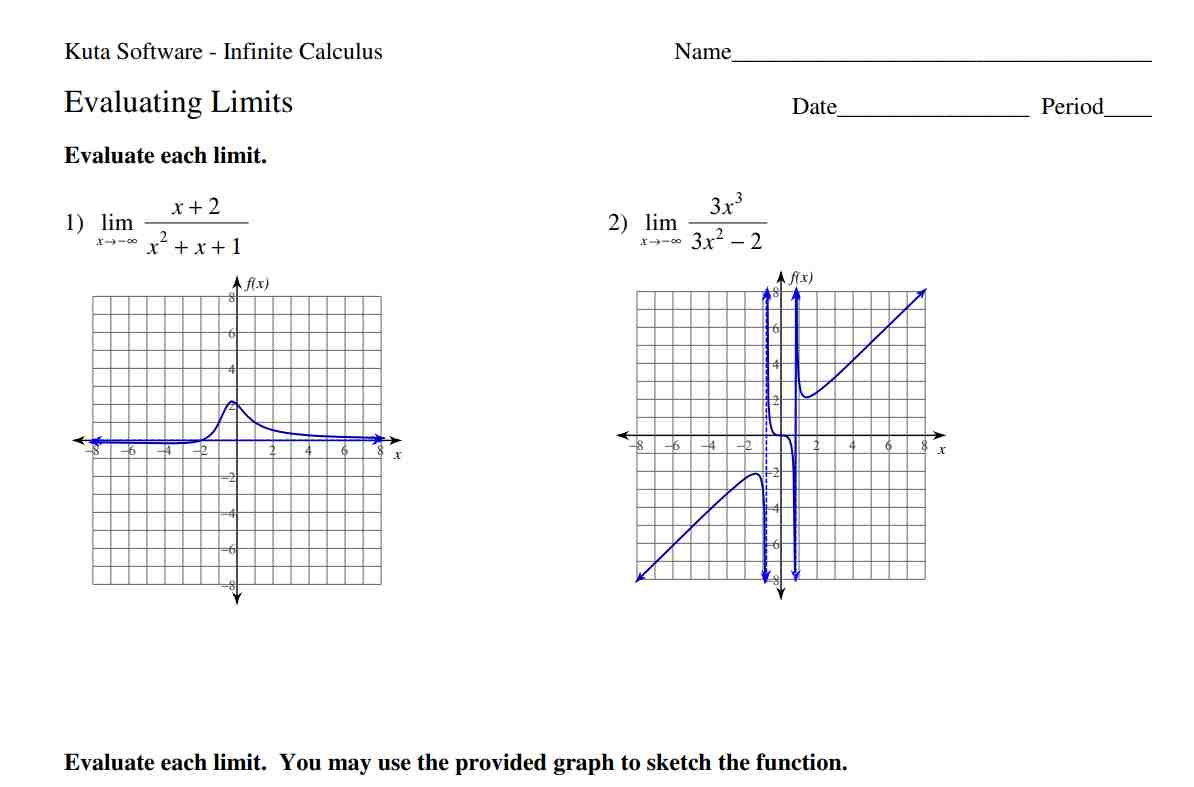
infinite calculus evaluating limits worksheet answers- (Paper 3)
For more papers FULL PLAYLIST :- | infinite calculus evaluating limits worksheet answers |
How to solve kuta software – infinite calculus evaluating limits worksheet answers
Step 1: Plug and Simplify
The first step is to substitute the value ‘a’ into the function ‘f(x)’ and simplify as much as possible. This might involve canceling out common factors or simplifying complex expressions.
Step 2: Check for Continuity
Next, check if the function ‘f(x)’ is continuous at ‘a.’ If it is, you can simply substitute ‘a’ into ‘f(x)’ to find the limit. If not, you’ll need to explore further.
Step 3: Factor and Cancel
If you encounter indeterminate forms like ‘0/0’ or ‘∞/∞,’ you’ll need to factor and cancel common terms to resolve these forms.
Step 4: Rationalize
For limits involving square roots or radicals, it’s often helpful to rationalize the expression by multiplying by the conjugate to simplify.
Kuta software – infinite calculus evaluating limits worksheet answers Complex Functions
Now that we’ve covered the basics, let’s move on to more complex functions and strategies for tackling them.
Trigonometric Limits
Using trigonometric identities and algebraic manipulation to simplify expressions when dealing with trigonometric functions.
Exponential and Logarithmic Limits
For limits involving exponential or logarithmic functions, consider applying L’Hôpital’s Rule or using the properties of these functions to simplify.
Special Techniques
In some cases, special techniques like Squeeze Theorem or the use of limit laws may be necessary to evaluate limits.
Practice Makes Perfect
The key to mastering limit problems is practice. Work through various examples, both simple and complex, to build your confidence and problem-solving skills.
Kuta software – infinite calculus evaluating limits worksheet answers Benefits of Using it
The worksheet offers several advantages for students:
Concept Clarity
Understanding limits at infinity is fundamental in calculus. This worksheet breaks down the concept into manageable steps, ensuring students grasp the core principles.
Variety of Problems
The worksheet provides a wide range of problems. Students can start with simpler ones and progress to more challenging ones, enhancing their problem-solving skills.
Step-by-Step Solutions
Each problem comes with a detailed solution. These solutions are designed to be clear and concise, making it easier for students to follow along and understand the process.
Multiple Methods
The worksheet introduces students to different methods for evaluating limits at infinity, allowing them to choose the approach that best suits their learning style.
How the Worksheet Helps with Concept Mastery
Understanding limits at infinity is crucial for success in calculus. This worksheet provides students with the foundation they need to excel in this area.
Preparation for Advanced Topics
A solid grasp of limits at infinity is often a prerequisite for advanced calculus topics, such as calculus of sequences and series. This worksheet serves as a stepping stone to these advanced concepts.
Conclusion
In conclusion, the Kuta Software – Infinite Calculus Evaluating Limits Worksheet is a valuable tool for students seeking to master the concept of evaluating limits at infinity.
Its structured approach, variety of problems, and step-by-step solutions make it an essential resource for both students and educators. By using this worksheet, students can build a strong foundation in calculus and prepare themselves for more advanced mathematical challenges.
Evaluating limits can be challenging, especially when faced with a worksheet like the one from Kuta Software’s “Infinite Calculus.” However, by following the steps outlined in this guide and practicing regularly, you can become proficient at solving even the most perplexing limit problems.
FAQs
What is the purpose of evaluating limits in calculus?
Evaluating limits helps us understand the behavior of functions as they approach specific points, which is crucial for various calculus applications.
Are there any shortcuts for solving limit problems?
While there are techniques like L’Hôpital’s Rule, there are no universal shortcuts. It’s essential to understand the fundamentals.
How can I check if a function is continuous at a particular point?
A function is continuous at a point if the limit as ‘x’ approaches that point equals the function’s value at that point.
What should I do if I encounter an indeterminate form in a limit problem?
In such cases, try factoring, canceling, or applying L’Hôpital’s Rule to resolve the indeterminate form.
Where can I find additional practice problems for evaluating limits?
You can find practice problems in calculus textbooks, online math resources, or ask your instructor for extra exercises.